It’s Fibonacci Day!
It’s Fibonacci Day!
0, 1, 1, 2, 3, 5, 8, 13, 21, 34, 55, 89, 144…
Wondering why I am placing the numbers so randomly? Are they random, though?
If you look close enough, there will soon appear a simple yet beautiful pattern.
The Fibonacci sequence is a series where each number is the sum of the two preceding ones. Starting at 0: add 1, you get 1. Add 1 and 1, you get 2. Then 2 and 3 make 5, and so it continues infinitely. While some writers start the sequence at 0 and 1, others prefer 1 and 1—or even Fibonacci’s own choice of 1 and 2. But that wouldn’t hurt our understanding of the basic idea anyway.
So, if you’ve just learned what the Fibonacci sequence is—congratulations! And if you already knew, congratulations to you too!
Why is today Fibonacci Day?
Here’s the fun part: today’s date, written in the mm/dd format, is 11/23. Notice the pattern? 1, 1, 2, 3—Fibonacci numbers!
That’s why today is celebrated as Fibonacci Day.
Who was Fibonacci?
A quick thanks to the mediaeval mathematician Mr Fibonacci (well, Fibonacci means “son of Bonacci” and his actual name is Leonardo Bonacci).
But a very interesting fact is, Fibonacci didn’t invent the Fibonacci numbers! Then why it is named after him, you might wonder.
Actually, the Fibonacci numbers were first described in Indian mathematics as early as 200 BC in work by Pingala.
Later, Italian mathematician Fibonacci—also known as the Leonardo of Pisa—was the one who introduced and popularised the sequence to Western European mathematics, back in 1202, in his book Liber Abaci (Latin for ‘The Book of Calculation’).
Now, I wouldn’t make this article math heavy; rather, let’s talk about two of the most fascinating topics Fibonacci numbers are related to: The Golden Ratio (I bet many of you have already heard about it!) and the Rabbit problem.
The Golden Ratio
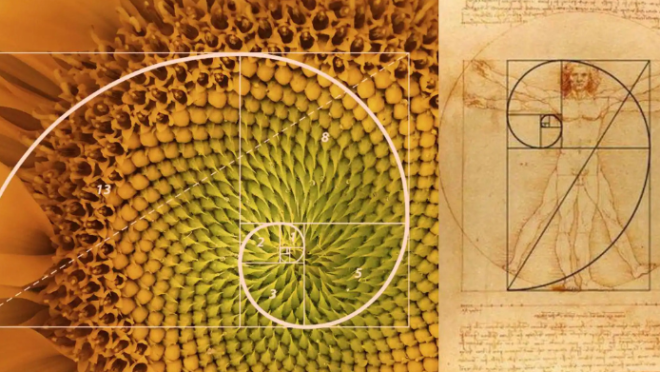
The Golden Ratio is what you get when you divide a number in the Fibonacci sequence by its predecessor. The further you go along, the closer the ratio gets to an irrational number called Phi (approximately 1.618033…). This number is so special that it’s often called “the most beautiful number in the universe.”
You can spot the Golden Ratio in nature—like the spiral of sunflower seeds or the way petals grow around a flower’s centre. It also appears in art, architecture, and design, adding a touch of harmony wherever it shows up.
The Rabbit Problem
In Liber Abaci, Fibonacci posed a puzzle: If a pair of rabbits is placed in a walled garden, and every month each pair produces another pair (starting from their second month), how many pairs of rabbits would there be in a year?
The answer unfolds into the Fibonacci sequence:
0, 1, 1, 2, 3, 5, 8, 13, 21, and so on.
The first month, there are no rabbits. By the second, a single pair. By the fourth month, there’s another pair, and by the sixth, the numbers start growing rapidly—each new number being the sum of the previous two. It’s a clever way to explain the sequence and its exponential growth.
Mathematics is beautiful; all you need to do is pause a bit and ponder.
Happy Fibonacci Day!


